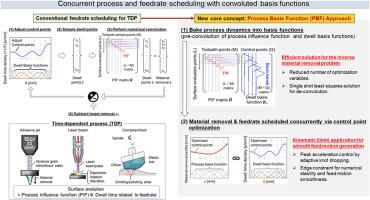
Non-traditional laser and fluid jet processes exhibit time-dependent material removal characteristics. The feedrate profile must be planned carefully along the toolpath for accurate surface profile generation while ensuring that the kinematic limits of machine tools are not violated. Conventional methods iteratively solve a deconvolution/convolution problem on the dwell-time density (reciprocal of the feedrate profile) that is computationally heavy, may leave significant residual processing errors, and even generate infeasible feed profiles with the manufacturing equipment. This paper proposes a novel approach that fully addresses the shortcomings above. Dwell-time density is first expressed as a continuous B-spline profile. The associated dwell basis functions (DBF) are convolved with the process influence function (PIF) to generate new process basis functions (PBF). This approach conveniently allows the posing of the problem as a concurrent linear least-squares problem on the control points shared by the DBFs and PBFs while ensuring the numerical stability of the solution and smoothness of the feed profile. To mitigate excessive acceleration peaks and any ringing effect around the edges of the toolpath, this paper also presents methodologies for stabilizing the scheduled feedrate profile by introducing knot vector adjustments (adaptive knot dropping) and linear edge constraints. The effectiveness of the proposed method is demonstrated and validated through simulation case studies and experimentally in fluid jet processing of precision optics. Results indicate that the proposed technique overcomes the limitations of conventional strategies and allows high-frequency surface components beyond the first zero-power frequency of the process footprint to be tracked while still generating a smooth feed profile within the acceleration limits of a machine tool. This ability stems from the localization characteristics associated with the basis functions. By improving the accuracy of high-frequency components, the proposed method exhibits the potential to fabricate topographies with sharper edges, which has been a challenge for conventional techniques.