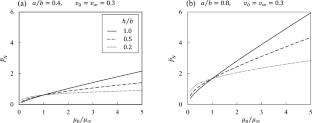
Direct bonding is an attractive technique to join material components without the use of intermediate adhesive medium. Usually, the bonding interface can experience high level of residual stress concentration due to entrapped nano-scale particulate contamination. Existing theoretical models are not capable of analyzing such residual stress concentration, since they fail to consider the localized material inhomogeneity formed between the bonding pairs as result of thermal and diffusion processes. This paper proposes a new theoretical model to analyze the residual stress concentration in the bonding interface with the consideration of localized material inhomogeneity. Following the idea of Selvadurai and Singh (Int. J. Fract. 25:69–77, 1984), the nano-scaled particulate contamination induced interfacial defect is simulated as a penny-shaped crack indented by a smooth rigid disc inclusion. This mode I crack-inclusion model is interpreted as a three-part mixed boundary value problem in the theory of elasticity, which is solved by a series expansion technique. Mathematical difficulties associated with modelling arbitrary localized material inhomogeneity are overcome by the use of the General Kelvin Solution (GKS) based method. Exact analytical solutions for the stress intensity factors (SIFs) and resultant force on the inclusion are obtained. Our results show that the inclusion-crack radius ratio and the localized material inhomogeneity can have significance effect on the residual stress concentration at the bonding interface.