Downstream Control on the Stability of River Bifurcations

Abstract

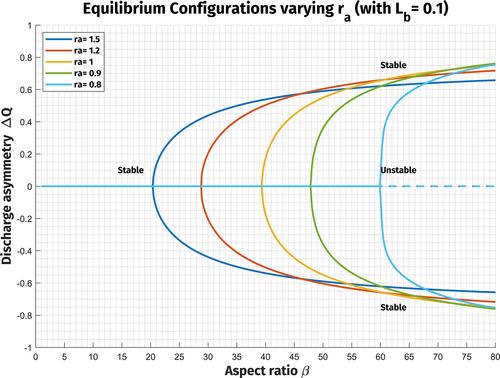
River bifurcations are prevalent features in both gravel-bed and sand-bed fluvial systems, including braiding networks, anabranches and deltas. Therefore, gaining insight into their morphological evolution is important to understand the impact they have on the adjoining environment. While previous investigations have primarily focused on the influence on bifurcation morphodynamics by upstream channels, recent research has highlighted the importance of downstream controls. In particular, in the case of rivers, current linear stability analyses for a simple bifurcation are unable to capture the stabilizing effect of branches length unless a confluence is added downstream. In this work, we introduce a novel theoretical model that effectively accounts for the effects of downstream branch length in a single bifurcation. To substantiate our findings, a series of fully 2D numerical simulations are carried out to test different branches lengths. Results from linear stability analysis show that bifurcation stability increases as the branches length decreases. These results are confirmed by the numerical simulations, which also show that, as the branch length tends to vanish, bifurcations are invariably stable. Finally, our results interestingly reveal that when a source of asymmetry, such as a free surface gradient or channel area advantage, is present at the node, there are scenarios in which the less-favored branch becomes dominant over the hydraulically favored branch.