Two-Dimensional Superconductivity and Anomalous Vortex Dissipation in Newly Discovered Transition Metal Dichalcogenide-Based Superlattices

Abstract

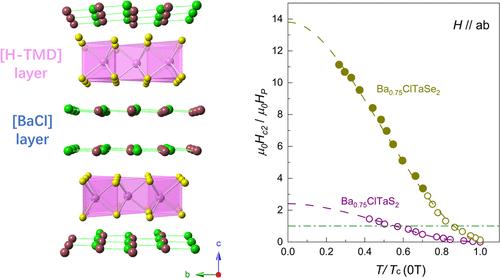
Properties of layered superconductors can vary drastically when thinned down from bulk to monolayer owing to the reduced dimensionality and weakened interlayer coupling. In transition metal dichalcogenides (TMDs), the inherent symmetry breaking effect in atomically thin crystals prompts novel states of matter such as Ising superconductivity with an extraordinary in-plane upper critical field. Here, we demonstrate that two-dimensional (2D) superconductivity resembling those in atomic layers but with more fascinating behaviors can be realized in the bulk crystals of two new TMD-based superconductors Ba_0.75ClTaS₂ and Ba_0.75ClTaSe₂ with superconducting transition temperatures 2.75 and 1.75 K, respectively. They comprise an alternating stack of H-type TMD layers and Ba–Cl layers. In both materials, intrinsic 2D superconductivity develops below a Berezinskii–Kosterlitz–Thouless transition. The upper critical field along the ab plane (𝐻||𝑎𝑏𝑐2$H_{c2}^{||ab}$$H_{c2}^{||ab}$) exceeds the Pauli limit (μ₀H_p); in particular, Ba_0.75ClTaSe₂ exhibits an extremely high 𝜇0𝐻||𝑎𝑏𝑐2${\mu }_0{H}_{c2}^{||ab}$${\mu }_0{H}_{c2}^{||ab}$≈ 14 μ₀H_p and a colossal superconducting anisotropy (𝐻||𝑎𝑏𝑐2$H_{c2}^{||ab}$$H_{c2}^{||ab}$/𝐻⊥𝑎𝑏𝑐2$H_{c2}^{\perp ab}$$H_{c2}^{\perp ab}$) of ∼150. Moreover, the temperature-field phase diagram of Ba_0.75ClTaSe₂ under an in-plane magnetic field contains a large phase regime of vortex dissipation, which can be ascribed to the Josephson vortex motion, signifying an unprecedentedly strong fluctuation effect in TMD-based superconductors. Our results provide a new path toward the establishment of 2D superconductivity and novel exotic quantum phases in bulk crystals of TMD-based superconductors.