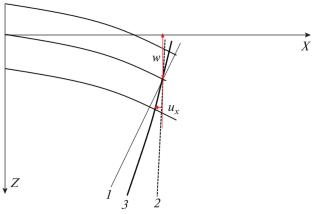
Abstract—Elastic bending stresses and deformations in the lithosphere are usually calculated based on the Kirchhoff–Love theory for thin plates. The criterion for its applicability is the smallness of the ratio of plate thickness to plate length. In oceanic plates, due to the buoyancy force of the mantle, the main deformations are not uniformly distributed along the plate but concentrate in the vicinity of the subduction zone. Therefore, the effective length of the bending part of the plate is a few fractions of its actual length, and the plate thinness criterion is partially violated. In this paper, we analyze the possibility of applying thick plate bending equations. The existing variational theories of 3D bending of thick plates are substantially more complicated than the Kirchhoff–Love theory, as they involve solving three differential equations instead of one, and have limited application due to their complexity. Since geophysical applications frequently use 2D models, in this paper we analyze in detail the potential and accuracy of the thick plate bending theory for 2D models. After the conversion to the 2D plane strain and plane stress approximation, the original 3D Reissner thick plate bending equations are written out in the form similar to the Kirchhoff equations with additive corrections and are supplemented with the explicit formulas for longitudinal displacement. The comparison of the analytical solutions of the 2D Reissner equations with the exact solutions shows that the 2D approximation only provides a correction for the plate deflection function. However, this correction refines the Kirchhoff–Love theory by almost an order of magnitude. At the same time, the solution of the equations in this case turns out to be almost as simple as the solution of the thin plate equations.