We investigate the Gross–Prasad conjecture and its refinement for the Bessel periods in the case of 
$(mathrm {SO}(5), mathrm {SO}(2))$. In particular, by combining several theta correspondences, we prove the Ichino–Ikeda-type formula for any tempered irreducible cuspidal automorphic representation. As a corollary of our formula, we prove an explicit formula relating certain weighted averages of Fourier coefficients of holomorphic Siegel cusp forms of degree two, which are Hecke eigenforms, to central special values of 
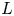$L$-functions. The formula is regarded as a natural generalization of the Böcherer conjecture to the non-trivial toroidal character case.
We categorify the inclusion–exclusion principle for partially ordered topological spaces and schemes to a filtration on the derived category of sheaves. As a consequence, we obtain functorial spectral sequences that generalize the two spectral sequences of a stratified space and certain Vassiliev-type spectral sequences; we also obtain Euler characteristic analogs in the Grothendieck ring of varieties. As an application, we give an algebro-geometric proof of Vakil and Wood's homological stability conjecture for the space of smooth hypersurface sections of a smooth projective variety. In characteristic zero this conjecture was previously established by Aumonier via topological methods.
We describe the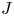 
$J$-invariant of a semisimple algebraic group 
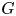$G$ over a generic splitting field of a Tits algebra of 
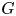$G$ in terms of the 
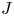$J$-invariant over the base field. As a consequence we prove a 10-year-old conjecture of Quéguiner-Mathieu, Semenov, and Zainoulline on the 
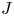$J$-invariant of groups of type 
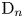$mathrm {D}_n$. In the case of type 
$mathrm {D}_n$ we also provide explicit formulas for the first component and in some cases for the second component of the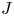 
$J$-invariant.
The loop space of a string manifold supports an infinite-dimensional Fock space bundle, which is an analog of the spinor bundle on a spin manifold. This spinor bundle on loop space appears in the description of two-dimensional sigma models as the bundle of states over the configuration space of the superstring. We construct a product on this bundle that covers the fusion of loops, i.e. the merging of two loops along a common segment. For this purpose, we exhibit it as a bundle of bimodules over a certain von Neumann algebra bundle, and realize our product fibrewise using the Connes fusion of von Neumann bimodules. Our main technique is to establish novel relations between string structures, loop fusion, and the Connes fusion of Fock spaces. The fusion product on the spinor bundle on loop space was proposed by Stolz and Teichner as part of a programme to explore the relation between generalized cohomology theories, functorial field theories, and index theory. It is related to the pair of pants worldsheet of the superstring, to the extension of the corresponding smooth functorial field theory down to the point, and to a higher-categorical bundle on the underlying string manifold, the stringor bundle.
京公网安备 11010802042870号
京ICP备2023020795号-1
扫码关注我们
求助内容：
应助结果提醒方式：