Clausen a prédit que le groupe des classes d’idèles de Chevalley d’un corps de nombres F apparaît comme le premier K-groupe de la catégorie des F-espaces vectoriels localement compacts. Cela s’est avéré vrai, et se généralise même aux groupes K supérieurs dans un sens approprié. Nous remplaçons F par une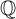 
$mathbb {Q}$-algèbre semi-simple, et obtenons le groupe des classes d’idèles noncommutatif de Fröhlich de manière analogue, modulo les éléments de norme réduite une. Même dans le cas du corps de nombres, notre preuve est plus simple que celle existante, et repose sur le théorème de localisation pour des sous-catégories percolées. Enfin, en utilisant la théorie des corps de classes, nous interprétons la loi de réciprocité d’Hilbert (ainsi qu’une variante noncommutative) en termes de nos résultats.
Clausen predicted that Chevalley’s idèle class group of a number field F appears as the first K-group of the category of locally compact F-vector spaces. This has turned out to be true and even generalizes to the higher K-groups in a suitable sense. We replace F by a semisimple 
$mathbb {Q}$-algebra and obtain Fröhlich’s noncommutative idèle class group in an analogous fashion, modulo the reduced norm one elements. Even in the number field case, our proof is simpler than the existing one and based on the localization theorem for percolating subcategories. Finally, using class field theory as input, we interpret Hilbert’s reciprocity law (as well as a noncommutative variant) in terms of our results.
We consider the notion of strong self-absorption for continuous actions of locally compact groups on the hyperfinite II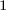
$_1$ factor and characterize when such an action is tensorially absorbed by another given action on any separably acting von Neumann algebra. This extends the well-known McDuff property for von Neumann algebras and is analogous to the core theorems around strongly self-absorbing C
$^*$-dynamics. Given a countable discrete group G and an amenable action 
$Gcurvearrowright M$ on any separably acting semifinite von Neumann algebra, we establish a type of measurable local-to-global principle: If a given strongly self-absorbing G-action is suitably absorbed at the level of each fibre in the direct integral decomposition of M, then it is tensorially absorbed by the action on M. As a direct application of Ocneanu’s theorem, we deduce that if M has the McDuff property, then every amenable G-action on M has the equivariant McDuff property, regardless whether M is assumed to be injective or not. By employing Tomita–Takesaki theory, we can extend the latter result to the general case, where M is not assumed to be semifinite.
京公网安备 11010802042870号
京ICP备2023020795号-1
扫码关注我们
求助内容：
应助结果提醒方式：